Z Modulo Pz

So the surjection pZ ppZ p. Before proving the proposition Im gonna remind you some fact proper to the finite groups.

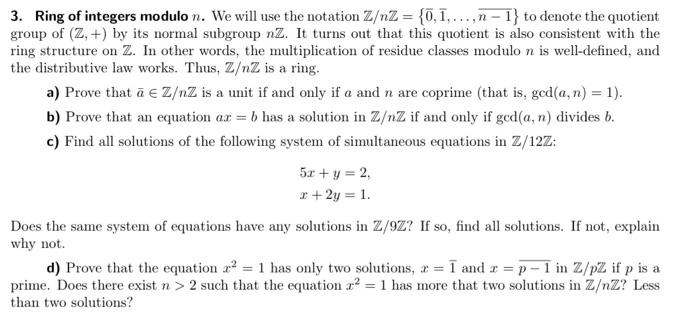
Solved 3 Ring Of Integers Modulo N We Will Use The Chegg Com

Then n a a and n.

Z modulo pz. In other words if we start with g and keep multiplying by g eventually we see every element. Modulo autocertificazioni assenze. Suppose α Q and.

List all elements of Z97Z of order. In other words the multiplicative order of a modulo n is the order of a in the multiplicative group of the units in the ring. If k Z kα 1 and there is a unique normalized cusp form F on X1Np of weight k ZpZ.

Autodichiarazion e per accesso visitatori. 244 Outline Some Background on Pseudo-Mersenne Numbers. M357 prim ZmZ Restklassen-Körper GLS 3x3 über Z3Z5Z7 Additions- Multiplikationstafel modulo m.

For a prime number p the group ZpZ is always cyclic consisting of the non-zero elements of the finite field of order p. Indeed a is coprime to n if and only if gcda n 1Integers in the same congruence class a b mod n satisfy gcda n gcdb n hence one is coprime to n if and only if the other is. Known facts about the quotient rings of the kind Z n I dont list all the characteristics of a ring just some ones.

Then there exists an M Z which depends only on p N and αwith the following property. Primitive root Artins conjecture Generalized Riemann Hypothesis Introduction For a prime p the multiplicative group ZpZ is cyclic. SMC AR30K-N03-Z regulator modular AR MASS PRO.

Modulo dichiarazione alunni fragili. ZpZ C p is a character. 3 is a generator of Z.

5 is a primitive root for Z97Z. Let τZpZ µQp denote the Teichmuller character. Modulo accettazionerinuncia iscrizione Strumento Musicale.

Units modulo a prime 1. On the other hand if a is a non-zero non-square then ab2 is a non-zero non-square for any b 0. WEIGHT SECTION MODULUS Moment of Inertia in4ft cm4m COATING AREA Flange tf in mm Wall tw in mm Pile lbft kgm Wall lbft2 kgm2 Elastic in3ft cm3m Plastic in3ft cm3m Both Sides ft2ft of single m m Wall Surface ft2ft2 of wall m m2 PZ 22 220 559 90 229 0375 950 0375 950 647 1369 403 600 220 1074 181.

You may refer to the tables in Rosens text to produce one primitive root but the rest should be computed by hand. We conclude that ZpZ is a field. There are many attractive theorems and conjectures.

One notices immediately that the. This is a legacy product and replaced by. Z Modular has designed a resource to help hospitals overcome capacity constraints.

We prove in B3. Let us now add a level of abstraction and consider for a prime p a2 b2 1 mod 4 the map λ. ZpZ Zia bi.

Every non-zero element of Z pZ is either a square or a non-square If a b2 is a non-zero square then as p 5 we have a b2 3b 52 4b 52 as a sum of two non-zero squares. In Rosens text there is a table of the smallest positive primitive roots for all primes less than 1000. Modulo adesione progetto Trinity.

What is Number Theory order. 24 alle Lösungsvektoren mod m 25 probe mod m Beispiel App. Modello delega ritiro alunni.

For the following primes p list all primitive roots of ZpZ. As a result the industry must find a way to get the work done with fewer hands. 3 The Structure of ZpZ The structure of prime power modulus unit groups begins simply with the case of prime modulus.

We have just proved that for a prime modulus pthe group of units ZpZ contains a primitive root. A p 19 b p 31 c p 43 2. The complete system of residues modulo a Gaussian prime abihas exactly Nabi a2 b2 elements.

America is short on skilled tradespeople. On Polynomial Modular Number Systems over ZpZ Jean Claude Bajard joint work with J er emy Marrez Thomas Plantard and Pascal V eron MACAO - Inria - University of Wollongong 2019 Mathematics and Algorithms for Cryptographic Advanced Objects. 3 is a generator of Z 4 since 3 1 3 3 2 1 are the units of Z 4.

They are commutative rings with unity. It obviously is a homomorphism because λr pλs p r abis abi. Please pull down this page to review full catalog specifications PDF.

Because there are only a nite number of values in ZpZ we know that there are only nitely many such points. Furthermore we get all the non-squares in this way. When ZnZ is cyclic its generators are called primitive roots modulo n.

21 qxy ax2 bxy cy2 dx ey f 0 mod p. In mathematics modular arithmetic is a system of arithmetic for integers where numbers wrap around when reaching a certain value called the modulusThe modern approach to modular arithmetic was developed by Carl Friedrich Gauss in his book Disquisitiones Arithmeticae published in 1801. It is a straightforward exercise to show that under multiplication the set of congruence classes modulo n that are coprime to n satisfy the axioms for an abelian group.

Kommutative Ringe sind genau dann Körper wenn jedes Element ausser 0 invertierbar ist. If G is a finite multiplicative group then every. When p is prime its proven that the group ZpZ is cyclic which means that it can be represented this way gk for k in 0p-1 so that each element of ZpZ will take the form.

Modulo richiesta uscita anticipata Scuola dellInfanzia. Description group Modulus Inertia flange web single 2 sides area in3ft in4ft inch inch inch inch lbsft lbsft2 ft2ft ft2ft cm3m cm4m mm mm mm mm kgm kgm2 m2m m2m PZ27 Hot rolled sheet piles Z 302 1842 1800 1200 0375 0375 405 2700 448 149 1624 25154 457 305 95 95 6026 13182 137 149. I want to prove that if p is prime then Z p is a field.

Series image - for reference only. To count the number of points in C qp we start by examining the equation these points satisfy. A commutative ring in which every nonzero element is a unit.

Order in the group of reduced residues modulo n. Yet demand for buildings continues to grow. In contrast Z8Z 1357 is a Klein 4-group and is not cyclic.

Recall that when pis a prime ZpZ is a eld ie. We will be interested in counting the number of elements in ZpZ of each allowable order. 0 n is the identity of addition or zero.

Quoteoff ZpZ ist wie du vielleicht weisst ein kommutativer Ring. Modular Solves the Construction Labor Shortage Problem. In number theory given an integer a and a positive integer n coprime to a the multiplicative order of a modulo n is the smallest positive integer k with.

PˇZpeZ to the multiplicative group map 1pZ p. A unit g Z n is called a generator or primitive root of Z n if for every a Z n we have g k a for some integer k. Now let Xbe the column vector hxyi and let Mbe the symmetric matrix M a b2 b2 c.

R p 7r abi. A e 1 is an isomorphism because the two nite groups have the same order and then A pe 1 is cyclic because Zpe 1Z is. Die Aussage ist also äquivalent dazu.

ZpeZ factors through the quotient of its domain pZ pby peZ p pZ p exp 1 pZ p pZ ppeZ p A e 1 Further pZ ppeZ pˇpZp eZ ˇZpe 1Z. Let Z p Z p Z be the quotient ring modulo p. A familiar use of modular arithmetic is in the 12-hour clock in which the.

2 11Z9 6 11Z. More precisely that ZpZ contains exactly p 1 distinct primitive roots. Quoteon 2008-01-08 2117 - natik IZ modulo pIZ ist Körper wenn p eine Primzahl ist Die Begründung dafür war aber nie dabei.

Modulo Sportello dascolto - consenso informato. Number theorists refer to any cyclic generator of this group as a primitive root modulo p.

Teori Bilangan

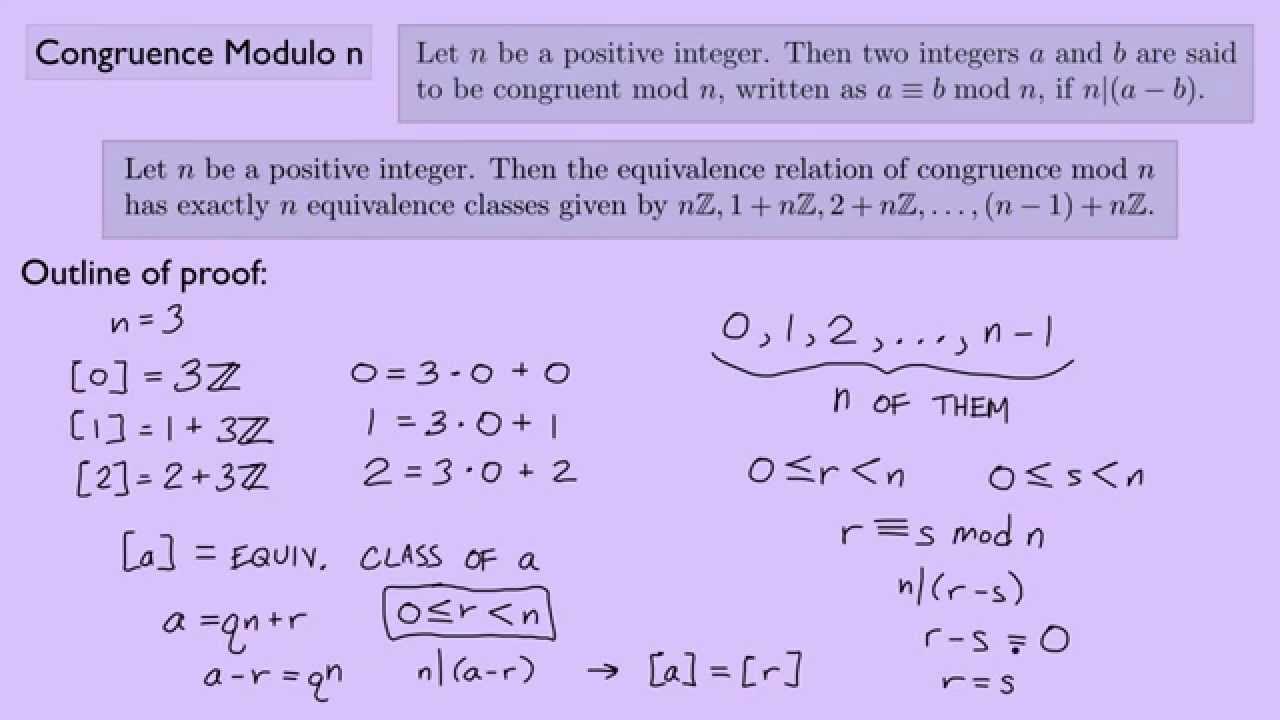
Abstract Algebra 1 Congruence Modulo N Youtube

Teori Bilangan

1 Sztuk Modul Am2302 Dht22 Cyfrowy Czujnik Temperatury I Wilgotnosci Pcb Z Kablem Dla Arduino Humidity Sensor Diy Electronic Kits Arduino

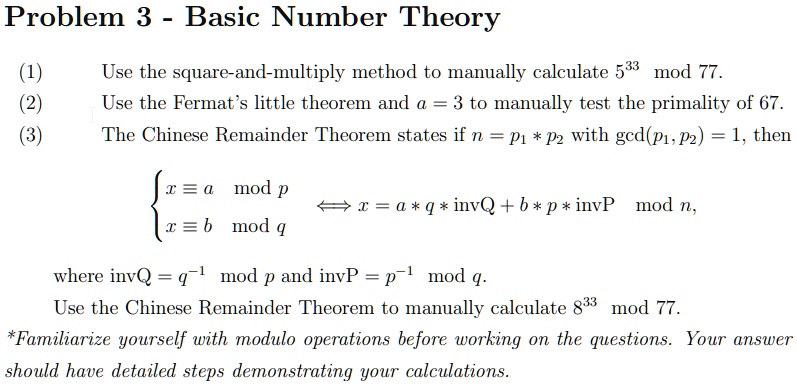
Solved Problem 3 Basic Number Theory Use The Square And Multiply Method To Manually Calculate 533 Mod 77 Use The Fermat S Little Theorem And A 3 To Manually Test The Primality Of